Theories behind a Chinese Reflective Solar Cooker

Li-Yan Zhu (朱立彦)

Introduction

A Chinese reflective solar cooker (Fig. 1) appears on Internet for years. I learned in Chinese websites that they were designed by a “Three-Circle Graphical Procedure (三圆作图计算法).” However no detail could be found on the Internet. I was quite thrilled last month, when T.H. Tan kindly sent me a paper by Chen Xiao-Fu (陈晓夫) and Ren Hong-Chen (任宏琛), both of the Chinese Agriculture Energy Association.

The paper was written in Chinese. It stated that the procedure was derived by “dozens of solar cooking specialists in China, through years of collaboration”, and that it “represents a significant advance in the theory of solar cooking.” In just one page, it described all three circles without proof or derivation.

Given equations of three circles, I was able to replicate the entire derivation. It is posted below. I hope that it inspires additional theoretical work in the field of solar cooking.

Fig. 1 Widespread use of the Chinese solar cooker

(Photos excerpted from various sites on the internet)

Assumptions

A parabolic reflector with a focal length (f) is mounted on an adjustable frame. All linear dimensions of the cooker are normalized against f. While the focal point F is fixed at a constant height H above the ground, the optical axis can be adjusted manually or automatically to track the sun. Elevation j of the sun is assumed to be in a predetermined range (a<j<b, typically between 25° and 70°).

A pot is mounted on a non-moving part of the frame. It has a flat, dark bottom that remains horizontal at all times. The center of this bottom surface is placed at the focal point F, to receive reflection from the reflector.

The reflector must meet three requirements:

Limited angle of incidence (q, typically 70°) at F;
No interference with the ground;
Desired sunlight collecting area.

Derivation

§1 Truncation by the Collection Cones

At any given elevation j, admissible reflection arriving at F is confined in a cone whose apex angle is 2q. This cone is called the collection cone.

Let Oxyz be a Cartesian coordinate system fixed to the reflector, where O is at the apex of the parabolic surface and z-axis coincides with the optical axis. Then the reflecting surface is a subset of

(1)

Reflection from an arbitrary point P(x, y, z) to the focal point F(0, 0, 1) is in the direction

(2)

For clarity assume that the yz-plane contains the ecliptic (i.e., the sun moves in the yz-plane). The axis of collection cone is in a direction

(3)

Lateral surface of the collection cone is defined by

(4)

where

(5)

Combine eqs. (2) through (5),

(6)

Introduce eqn. (1) into (6),

(7)

Rearrange: ,

(8)

Eliminate z by introducing eqn. (1) into eqn. (8). Then complete the square:

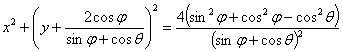(9)

Equation (9) defines a cylinder parallel with the z-axis. In the xy-plane, eqn. (9) defines a projection of the reflector. It is a circle centered at (0, y_j), and radius r_j, where

(10)

(11)

The circle is called the j-circle. As elevation j of the sun increases from a to b, the circle moves up the y-axis while its radius decreases. The area admissible at all intended elevation j is outlined by two circles, viz. the a-circle and the b-circle.

§2 Truncation by the Ground

The reflector is more likely to interference with the ground when the sun is low. Thus it suffices to consider the extreme case of j=a. Adding a subscript a to eqn. (3), the axis of collection cone is in the direction:

(12)

Let this axis intersect the ground at point Q. Then

(13)

An arbitrary point P(x, y, z) is on the ground if and only if

(14)

i.e.,

(15)

Expand, and rearrange:

(16)

Introduce eqn. (1) into (16). Then divide by sina,

(17)

Complete the square. Then use trigonometry identity and rearrange

(18)

In the xy-plane, eqn. (18) defines a circle centered at (0, y_H), and radius r_H, where

(19)

(20)

It will be called the H-circle in the sequel. The smaller is H, the larger is the H-circle, and the skinnier is the reflector.

§3 Area of Sunlight Collection

The sunlight collecting area is the intersection of a and b circles, less the intersection of a and H circles (Fig. 2). According to Chen et al., all three circles are to be drawn on a graph paper. Then evaluate the collecting area by counting the number of squares. Adjust the focal length f until desired collection area is achieved.

I do not know why Chen et al. did not provide an analytical solution for the area. It is more expedient than counting the squares. Consider two arbitrary intersecting circles U and V. Let their radii be u and v, respectively; the distance between their centers be d; and the area of their intersection be A_UV. Then

, (21)

, (22)

(23)

The actual (not normalized) collection area is

(24)

Both terms on the right-hand side of eqn. (24) are calculated by eqs. (21) through (23) in identical manner, with the subscripts identifying intersecting circles. Any desired collection area A can be attained by adjusting f along, without changing the reflector shape (i.e., the parameter H).

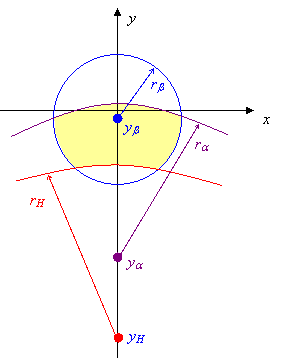Fig. 2 Three circles in the xy-plane define the collection area (shaded)

Comments

The three-circle design method is elegant and practical. It certainly has advanced the course of solar cooking in China. The method itself is flawless. However a constrain imposed on the cooker, hence an assumption in the derivation, is not immutable.

The assumption to be discussed is that heat should be applied to the center of the bottom surface. This requirement is not without merit. Normally a solar cooker had few believers at its debut. Thus it was beneficial to demonstrate the intense heat at the focus. Most Chinese solar cooker manufacturers still strive for the bragging right of their “hot spot”. One advertisement touts 800 °C. It is also beneficial for villagers to cook much the same way their did on conventional stoves. In short, the “hot spot” helped solar cooker to gain acceptance from desperately fuelicken farmers.

However cooking in the hot sun can be quite uncomfortable. Glare from the reflector may strain or even damage eyes. Spillage can tarnish the reflector. Uneven heating associated with a sharp focus is also undesirable for most cooking tasks (Even on traditional stoves or range tops, cookware that promises uniform heating commands a premium). Therefore solar cooker with a “hot spot” is likely to lose market as the standard of living improves.

A “hot spot” on the bottom surface also begets a structure disadvantage. Compared with solar cookers that heat in all directions (such as SK-14 and my unattended parabolic cooker), the Chinese cooker requires a much larger focal length. Thus angular tolerance of its reflecting surface is much tighter. Not only the reflector must be made more precise, it must be re-aligned with the sun more often. Specifically, my unattended reflective cooker cooks up to 2 hours without re-alignment. The Chinese cooker needs to be re-aligned every ten to fifteen minutes.

Summary

The Chinese have developed a parabolic solar cooker with a hot spot at the bottom of a cookware. The reflector is contoured such that the angle of incidence on the cookware never exceeds a predetermined value (~70°). A graphical design procedure was also developed for the reflector contour. The solution consists of three circles. Two of the circles are due to the angle of incidence. The third is due to the height of support.